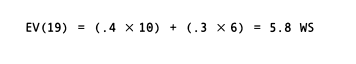Highest Future Value
This article was written by Tom Hanrahan
This article was published in 2003 Baseball Research Journal
The good hot stove question is: What ballplayer, at the peak of his career, could you most solidly say, “I wouldn’t trade him for anybody“?
Stated another way, if we take all major leaguers and track their careers as they progress (with no future knowledge), which one would have been perceived as having the highest future value (or value in a potential trade) of all time. It would have to be a player early enough in his career that he still had many years of service remaining, but also far enough into his career that he had established a high level of performance.
The metric I used to answer this question was the Bill Jamesian uber-stat: Win Shares. In fact, this is precisely the type of query that Win Shares is qualified to answer. As a measure, it is comprehensive, easy to use, and well-accepted in sabermetric circles.
For those unfamiliar with Win Shares (WS), it is an attempt to take all of a player’s contributions in a season and assign them a value in terms of how many wins he contributed to his team. Offensive rates (on base average, slugging, stolen base success), playing time, fielding stats, defensive positions, and clutch play are all taken into account. The denomination of WS is actually “wins divided by 3”.
As examples, Babe Ruth has the highest total of WS ever; with 758 WS, he was worth 758 ÷ 3 = 253 wins in his career to his teams, above a poor replacement outfielder/pitcher. In 2001, when Barry Bonds hit 73 home runs and set many other records, he collected 54 WS, which is one of the highest single-season totals ever. A complete season by-season listing of the WS all of these players earned can be found in the book entitled Win Shares.
To measure a player’s current value or what I will call established value (EV), I used a weighted average of WS over the most recent four years: 40% of the current year, 30% the previous year, 20% of the year before that, and 10% in year minus three. I then researched every hitter I could find who had strong seasons at young ages to see who had the highest EV at a given age.
For this exercise, I did not compare pitchers with hitters; I reasoned that the comparatively larger number of career-threatening injuries to young pitchers would never allow a bright shining light such as a young Doc Gooden to be thought of as even trade bait for an equally young and brilliant Alex Rodriguez. So, my going-in assumption was that no pitcher was ever perceived as so good and such a horse and so injury-free that he might compare with a young, established superstar hitter.
Many players, even future Hall of Famers, did not play in the majors prior to age 20 or 21. Since a player can easily earn 10 to 15 WS in a season by playing full-time even when not playing very well, I decided to assign a minimum number of WS to any early missing player-seasons, assuming that if the major league club had called up the player, he would have performed at a certain level. This keeps the early list from being dominated by those players who were fortunate to have been brought up to the big leagues at 18 or 19.
It still does not adequately make up for those who served in the military at an early age; Willie Mays may have established one of the top EVs at ages 23 through 25 if he hadn’t missed time. The minimum assigned WS are as follows: 6 at age 18, 10 at age 19, 12 at age 20, and 14 at age 21 and following.
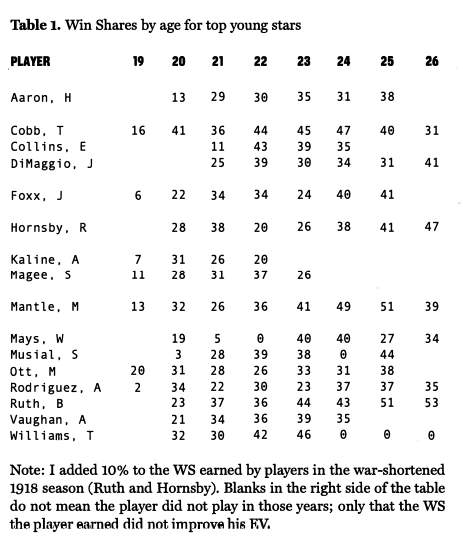
Table 1 shows the WS earned by age for each player who had one of the six highest established values at some point prior to age 27. There were some players who played in the majors at age 18, but since none of them in this table earned more than 6 WS at that age, the “age 18” column was dropped. Other players did earn more than 6 WS at age 18, but they did not become big enough stars later on, and so did not factor into the analysis. Nineteenth-century performances were not considered.
Traditionally, a player’s baseball age is how old he was on July 1 of the year. Later in the analysis, I will note which players are hurt or helped by this arbitrary date, and who else might have had the highest EV if another date was used.
Example: Rogers Hornsby. He did not play MLB at age 18 and earned no WS for his few games at age 19. So, at age 19, his Established Value is computed as
Where 10 and 6 are the minimum WS assigned for ages 18 and 19.
At age 20 (the year 1916), Hornsby suddenly blossomed. He was one of the best hitters in the league, finishing fourth in batting, slugging more than 100 points higher than any of his teammates, and split time between SS and 3B. For this, he earned 28 WS. His EV at age 20 is EV(20) = .4 x 28 + .3 x 10 + .2 x 6 = 15.4.
At age 21, Hornsby was the St. Louis Cardinals’ full-time shortstop and led the league in slugging, total bases, OPS, and triples. He earned 37 WS. His EV(21) = .4 x 37 + .3 x 28 +.2 x 10 + .1 x 6 = 25.8. This is the second-highest EV(21) ever.
To put 25.8 WS in perspective, Bill James has written that 30 WS is an “MVP candidate season.” So here we have a 21-year-old who has established that he is already almost a consistent MVP candidate. Probably not someone you are going to trade for most any other player.
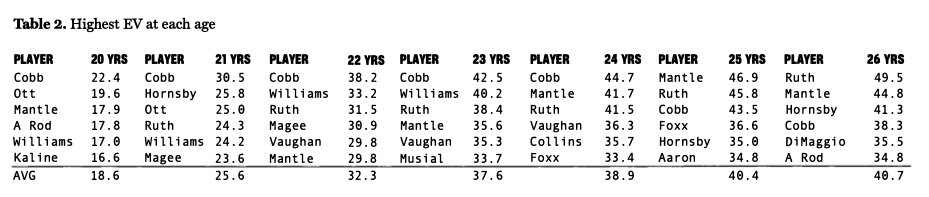
Table 2 shows the six highest EV at each age from 20 through 26. No attempts have been made to attempt to adjust for the vast differences in playing conditions, quality of play, or schedule. It should be noted that only four of the 15 players mentioned in the table were youngsters in the post-integration era (Mickey Mantle, Henry Aaron, Al Kaline, and Alex Rodriguez). One player who is not listed on the table, but is on a rapid ascent toward young greatness, is Albert Pujols. After the 2003 season, he has an EV(23) of 31.8, having earned 29, 32, and 41 WS in his first three seasons. Another season like the others will put Mr. Pujols in elite company
The players listed in italics are those whose birth days were in the months of January through June. They are on the young side of each year in question. Ty Cobb has the highest EV(21) of 30.5 WS, but Ty was really an “old” 21; he turned 22 shortly after the season ended. Mel Ott (born in March) was actually about three months younger than Cobb at the same “age.” If I had chosen January 1 instead of July 1 as the cutoff date, Ott would be at the top of the age 21 group.
(Click image to enlarge)
Table 2 shows a sharp rise in EVs in general from ages 20 through 23, and then small increases after that. Babe’s EV(26) of 49.5 EV was the highest he had ever reached. It is not the highest EV of all time, however. Honus Wagner earned 46, 43, 44, and 59 WS from ages 31 through 34, giving him an EV(34) of an even 50.0. The highest “old player” EV is owned by Barry Bonds, who after the 2003 season has an EV(38) of 44.3.
How should we turn EV into “future value”? Future value should simply be a function of EV and age. I say simply because that is only two parameters, but the function would be difficult to agree on. Extensive research would have to be done on MLB player success and the aging process. Rather than attempting to create one grand number that attempts to definitively answer the question, I would encourage the reader to eyeball the figures in Table 2 with me.
As good as the young Mel Ott was at ages 20 and 21, it does not seem reasonable to conclude that a manager would refuse hypothetically to trade him for one of the vastly superior players who were only a few years older. The real battle is between the young Ty Cobb, ages 22 through 24, and the more established Babe Ruth. The peak in Cobb’s EV curve occurs at age 22. He had just won three consecutive batting titles (1907 through 1909), as well as having led the league in slugging, hits, total bases, and RBI three years in a row.
In contrast, the Babe continued to add significantly to his established value by whacking 50+ home runs (more than most teams) and shattering numerous other records at ages 25 and 26 (1920 and 1921). How should we compare Cobb’s EV(22) of 38.2 with Ruth’s EV(26) of 49.5, remembering that Ruth’s birthday was two months after Cobb’s, giving him a small age disadvantage?
Advantage for the Babe: His EV(26) is 30% higher than Ty’s EV(22).
Advantages for Ty: He was almost four years younger, which meant likely a longer career ahead of him, plus much more room for the growth that a 22-year old player would expect to have.
It is clear to me that the combination of these two factors is larger than the difference in their established values.
Conclusion
If Win Shares were the perfect value-measurement tool, and if there were no subjective factors to consider, and if no adjustments need to be made for changes in quality of play over time, then Tyrus Raymond Cobb, age 22 in the fall of 1909, would appear to have great est future value than any player at any point in major league history. Conclusions relevant to baseball today: Alex Rodriguez has been the best young player to grace the major leagues in at least the past 40 years-but watch out for Albert Pujols.
TOM HANRAHAN is an avionics systems engineer at the Naval Air Warfare Center in Maryland. He used to have other hobbies until baseball research and his family absorbed his time.